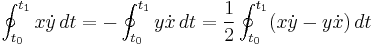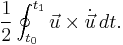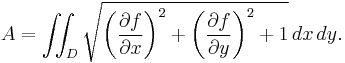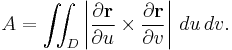Area
Area is a quantity expressing the two-dimensional size of a defined part of a surface, typically a region bounded by a closed curve. The surface area of a 3-dimensional solid is the total area of the exposed surface, such as the sum of the areas of the exposed sides of a polyhedron. Area is an important invariant in the differential geometry of surfaces.[1]
Contents |
Units
Units for measuring area, with exact conversions, include:
- square metre (m2)
- are (a) = 100 square metres (m2)
- hectare (ha) = 100 ares = 10000 square metres
- square kilometre (km2) = 100 hectares = 10000 ares = 1000000 square metres
- square megametre (Mm2) = 1000000000000 square metres
- square foot = 144 square inches = 0.09290304 square metres
- square yard = 9 square feet = 0.83612736 square metres
- square perch = 30.25 square yards = 25.2928526 square metres
- acre = 10 square chains = one furlong by one chain = 160 square perches = 4840 square yards = 43560 square feet = 4046.8564224 square metres
- square mile = 640 acres = 2.589988110336 square kilometres
- Kanal (unit) = 0.125 acres.
Formulae
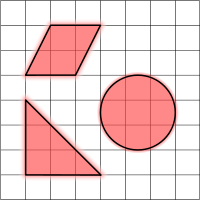
| Shape | Formula | Variables |
|---|---|---|
| Regular triangle (equilateral triangle) | 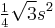 |
 is the length of one side of the triangle. is the length of one side of the triangle. |
| Triangle |  |
 is half the perimeter, is half the perimeter,  , ,  and and  are the length of each side. are the length of each side. |
| Triangle | 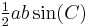 |
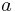 and and  are any two sides, and are any two sides, and  is the angle between them. is the angle between them. |
| Triangle |  |
 and and 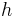 are the base and altitude (measured perpendicular to the base), respectively. are the base and altitude (measured perpendicular to the base), respectively. |
| Square | 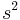 |
 is the length of one side of the square. is the length of one side of the square. |
| Rectangle | 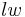 |
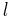 and and 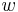 are the lengths of the rectangle's sides (length and width). are the lengths of the rectangle's sides (length and width). |
| Rhombus | 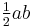 |
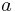 and and  are the lengths of the two diagonals of the rhombus. are the lengths of the two diagonals of the rhombus. |
| Parallelogram | 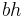 |
 is the length of the base and is the length of the base and 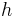 is the perpendicular height. is the perpendicular height. |
| Trapezoid | 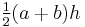 |
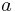 and and  are the parallel sides and are the parallel sides and 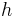 the distance (height) between the parallels. the distance (height) between the parallels. |
| Regular hexagon | 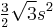 |
 is the length of one side of the hexagon. is the length of one side of the hexagon. |
| Regular octagon | 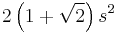 |
 is the length of one side of the octagon. is the length of one side of the octagon. |
| Regular polygon |  |
 is the sidelength and is the sidelength and 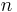 is the number of sides. is the number of sides. |
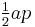 |
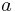 is the apothem, or the radius of an inscribed circle in the polygon, and is the apothem, or the radius of an inscribed circle in the polygon, and  is the perimeter of the polygon. is the perimeter of the polygon. |
|
| Circle | 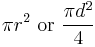 |
 is the radius and is the radius and 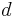 the diameter. the diameter. |
| Circular sector | 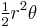 |
 and and 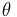 are the radius and angle (in radians), respectively. are the radius and angle (in radians), respectively. |
| Ellipse | 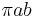 |
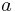 and and  are the semi-major and semi-minor axes, respectively. are the semi-major and semi-minor axes, respectively. |
| Total surface area of a Cylinder | 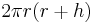 |
 and and 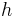 are the radius and height, respectively. are the radius and height, respectively. |
| Lateral surface area of a cylinder | 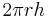 |
 and and 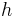 are the radius and height, respectively. are the radius and height, respectively. |
| Total surface area of a Cone | 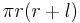 |
 and and 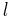 are the radius and slant height, respectively. are the radius and slant height, respectively. |
| Lateral surface area of a cone | 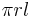 |
 and and 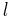 are the radius and slant height, respectively. are the radius and slant height, respectively. |
| Total surface area of a Sphere | 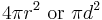 |
 and and 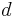 are the radius and diameter, respectively. are the radius and diameter, respectively. |
| Total surface area of an ellipsoid | See the article. | |
| Total surface area of a Pyramid | 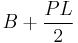 |
 is the base area, is the base area,  is the base perimeter and is the base perimeter and 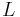 is the slant height. is the slant height. |
| Square to circular area conversion | 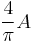 |
 is the area of the square in square units. is the area of the square in square units. |
| Circular to square area conversion | 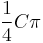 |
 is the area of the circle in circular units. is the area of the circle in circular units. |
The above calculations show how to find the area of many common shapes.
The area of irregular polygons can be calculated using the "Surveyor's formula".[2]
Additional formulae
Areas of 2-dimensional figures
- a triangle:
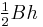 (where B is any side, and h is the distance from the line on which B lies to the other vertex of the triangle). This formula can be used if the height h is known. If the lengths of the three sides are known then Heron's formula can be used:
(where B is any side, and h is the distance from the line on which B lies to the other vertex of the triangle). This formula can be used if the height h is known. If the lengths of the three sides are known then Heron's formula can be used: 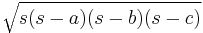 (where a, b, c are the sides of the triangle, and
(where a, b, c are the sides of the triangle, and  is half of its perimeter) If an angle and its two included sides are given, then area=absinC where C is the given angle and a and b are its included sides. If the triangle is graphed on a coordinate plane, a matrix can be used and is simplified to the absolute value of (x1y2+ x2y3+ x3y1 - x2y1- x3y2- x1y3) all divided by 2. This formula is also known as the shoelace formula and is an easy way to solve for the area of a coordinate triangle by substituting the 3 points, (x1,y1) (x2,y2) (x3,y 3). The shoelace formula can also be used to find the areas of other polygons when their vertices are known. Another approach for a coordinate triangle is to use Infinitesimal calculus to find the area.
is half of its perimeter) If an angle and its two included sides are given, then area=absinC where C is the given angle and a and b are its included sides. If the triangle is graphed on a coordinate plane, a matrix can be used and is simplified to the absolute value of (x1y2+ x2y3+ x3y1 - x2y1- x3y2- x1y3) all divided by 2. This formula is also known as the shoelace formula and is an easy way to solve for the area of a coordinate triangle by substituting the 3 points, (x1,y1) (x2,y2) (x3,y 3). The shoelace formula can also be used to find the areas of other polygons when their vertices are known. Another approach for a coordinate triangle is to use Infinitesimal calculus to find the area. - a simple polygon constructed on a grid of equal-distanced points (i.e., points with integer coordinates) such that all the polygon's vertices are grid points:
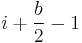 , where i is the number of grid points inside the polygon and b is the number of boundary points. This result is known as Pick's theorem.
, where i is the number of grid points inside the polygon and b is the number of boundary points. This result is known as Pick's theorem.
Area in calculus

- the area between the graphs of two functions is equal to the integral of one function, f(x), minus the integral of the other function, g(x).
- an area bounded by a function r = r(θ) expressed in polar coordinates is
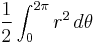 .
. - the area enclosed by a parametric curve
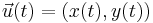 with endpoints
with endpoints  is given by the line integrals
is given by the line integrals
(see Green's theorem)
- or the z-component of
Surface area of 3-dimensional figures
- cube:
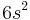 , where s is the length of the top side
, where s is the length of the top side - rectangular box:
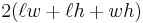 the length divided by height
the length divided by height - cone:
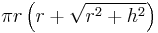 , where r is the radius of the circular base, and h is the height. That can also be rewritten as
, where r is the radius of the circular base, and h is the height. That can also be rewritten as 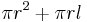 where r is the radius and l is the slant height of the cone.
where r is the radius and l is the slant height of the cone. 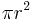 is the base area while
is the base area while 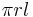 is the lateral surface area of the cone.
is the lateral surface area of the cone. - prism: 2 × Area of Base + Perimeter of Base × Height
General formula
The general formula for the surface area of the graph of a continuously differentiable function 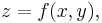 where
where 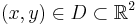 and
and  is a region in the xy-plane with the smooth boundary:
is a region in the xy-plane with the smooth boundary:
Even more general formula for the area of the graph of a parametric surface in the vector form 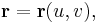 where
where  is a continuously differentiable vector function of
is a continuously differentiable vector function of 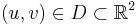 :
:
Minimization
Given a wire contour, the surface of least area spanning ("filling") it is a minimal surface. Familiar examples include soap bubbles.
The question of the filling area of the Riemannian circle remains open.
See also
References
Notes
- ↑ 1.0 1.1 do Carmo, Manfredo. Differential Geometry of Curves and Surfaces. Prentice-Hall, 1976. Page 98.
- ↑ http://www.maa.org/pubs/Calc_articles/ma063.pdf